Averaging time

The appropriate averaging time to be used can be determined by the use of an ogive function (Foken and Wichura, 1996). This function is defined as the cumulative integral of the cospectra beginning with the highest frequencies. The cospectra of scalar and is defined as follows: let F and F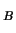 be the discrete Fourier transform of the simultaneously measured scalars and 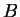, respectively. Let and 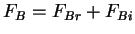, where subscripts and 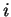denote real and imaginary parts of the discrete Fourier transform, respectively. The cospectrum is defined as

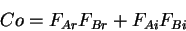$$Co=F_{Ar}F_{Br}+F_{Ai}F_{Bi}$$ (2.42)

(Stull, 1988). It is important to note that the sum over frequency of all cospectral amplitudes, 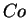, equals the covariance between and 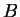. The ogive function has a form of

$$Og_{\overline{A'B'}}=\int ^{n_{0}}_{\infty }Co_{\overline{A'B'}}\left( n\right) dn\: ,$$ (2.43)

for any scalar flux , where denotes natural frequency and 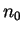is the lowest frequency contributing to the integral. This ogive function converges to a constant value at a frequency which could be converted to the averaging time.

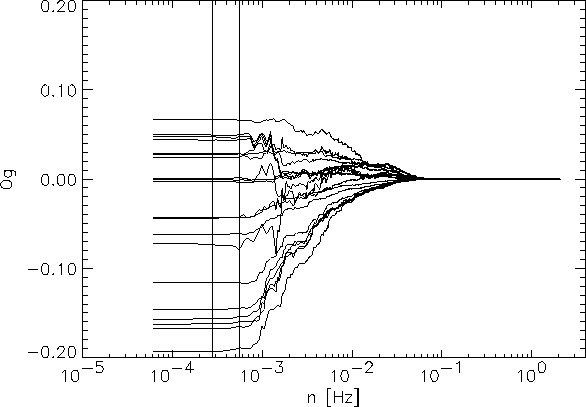
Figure: Carbon dioxide ogives (Og) based on 4.5 h periods, during 5 August, 1997. The vertical lines correspond to time periods of 1 and 0.5 h.

Figure shows the ogive functions for one day based on continuous 4.5 hours long time series. It can be seen that 0.5 hours averaging time is neary appropriate, but for precision 60 minutes averaging time was chosen to determine turbulent fluxes. We should note that closer to the surface 30 min averaging time is widely used in micrometeorology. Since turbulent scales become larger if we move away from the surface, a longer averaging time is required to resolve the low frequency part of the fluctuations which contributes to the turbulent transport. Also, the ogives show that 4 Hz sampling frequency is more than enough to resolve the high frequency scales contributing to the turbulent transport at the height of the measurement.